Differentiation of xcosx
Differentiate each of the following from first principle: x cos x. Differentiate each of the following from first principle: cos x x, differentiation of xcosx. Differentiate each of the following from first principle: x sin x.
The derivative of xcos x is equal to cosx — xsinx. The function xcos x is the product of x with its cosine. In this article, we will learn how to find the differentiation of xcos x using the following methods:. In the next two sections, we will prove this formula using the product rule of derivatives and the first principle of derivatives, that is, the definition of limits. Hence, the derivative of x cos x is cos x — x sin x obtained using the product rule of derivatives. Also Read:. Derivative of sin3x : The derivative of sin3x is 3cos3x.
Differentiation of xcosx
.
In this article, differentiation of xcosx, we will learn how to find the differentiation of xcos x using the following methods:. In this article, we will learn how to find the differentiation of xcos x using the following methods: Product rule of derivatives First principle of derivatives.
.
The derivative of xcos x is equal to cosx — xsinx. The function xcos x is the product of x with its cosine. In this article, we will learn how to find the differentiation of xcos x using the following methods:. In the next two sections, we will prove this formula using the product rule of derivatives and the first principle of derivatives, that is, the definition of limits. Hence, the derivative of x cos x is cos x — x sin x obtained using the product rule of derivatives. Also Read:. Derivative of sin3x : The derivative of sin3x is 3cos3x. Derivative of 1 : The derivative of 1 is zero.
Differentiation of xcosx
Learn how to calculate the derivative of a xcos x by first principle with easy steps. Also verify the derivative of xcos x by using chain rule and quotient rule. Derivatives have a wide range of applications in almost every field of engineering and science.
Express yourself song
Differentiate each of the following from first principle: e 3 x. View Solution. Video Solution. Differentiate each of the following from first principle: xcosx. Differentiate each of the following from first principle: -x. In this article, we will learn how to find the differentiation of xcos x using the following methods: Product rule of derivatives First principle of derivatives. Differentiate each of the following from first principle: xsinx. Differentiate each of the following from first principle: x cos x. Differentiate each of the following from first principle: xsinx Differentiate each of the following from first principle: x sin x. Was this answer helpful? Thus, the derivative of xcos x is equal to cosx-xsinx and this is obtained by the first principle of differentiation. We know that the derivative of a function f x by the first principle, that is, by the limit definition is given as follows.
One of the most important types of motion in physics is simple harmonic motion, which is associated with such systems as an object with mass oscillating on a spring.
The derivative of xcos x is equal to cosx — xsinx. Was this answer helpful? Share via:. Differentiate each of the following from first principle: xcosx Derivative of sin3x : The derivative of sin3x is 3cos3x. Q2: What is the derivative of xsinx? Differentiate each of the following from first principle: xsinx. Also Read:. Answer: The derivative of xcosx is equal to cosx-xsinx. The function xcos x is the product of x with its cosine. Differentiate each of the following from first principle: -x

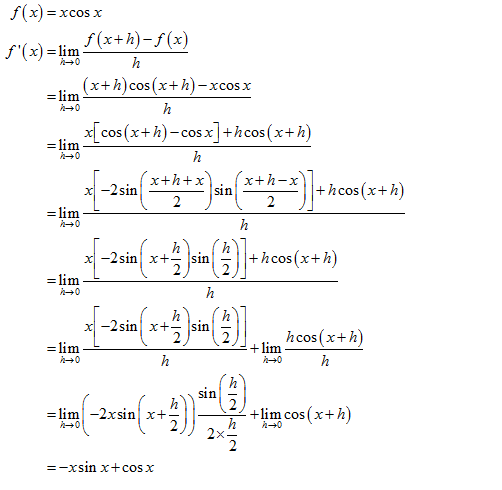
How will order to understand?
It is a pity, that I can not participate in discussion now. It is not enough information. But this theme me very much interests.
Charming question