Relationship between faces edges and vertices

Every geometric shape is composed of different parts such as vertices, faces, edges. We come across different objects with rectangular faces, circular faces, cubic faces, diamond faces, triangular faces, etc. We also know many objects that have sharp corners and edges. We will learn about vertices, faces, edges of different plane shapes and geometric shapes.

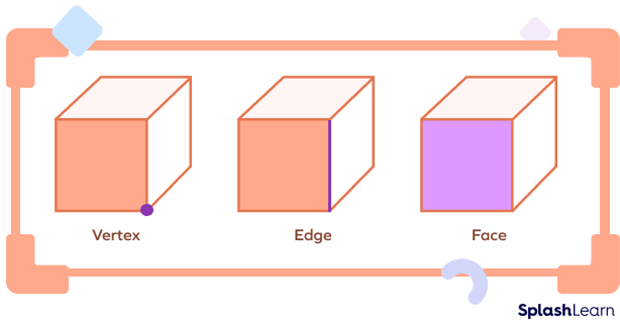
In three dimensional geometry we come across many solid shapes like sphere, cubes, cuboids, pyramids and so on. All these solid shapes are said to be made of plane or curved surfaces meeting at edges and vertices. The corner of the object is called a vertex. The flat surfaces are known as faces, and the straight lines that connect two faces are called edges. Each 3D shape has a different number of corners, flat surfaces, and straight lines. In this maths article, we shall learn about the faces, edges, and vertices of different 3d shapes in detail.

Relationship between faces edges and vertices

Here we will learn about faces, edges and vertices including how to calculate the number of vertices, edges and faces of a 3D shape, and how to classify polyhedrons given the number of faces, edges and vertices. To calculate the number of faces, edges and vertices of a 3D shape, we need to count the number of each using the 3D object. Note, you need to be able to visualise the 3D object, you may not be given the shape to help you. For example, a cube has 6 vertices, 12 edges and 6 faces. Below is a diagram of common 3D shapes split into polyhedra and non-polyhedra along with the number of vertices, edges and faces. Some of the most famous polyhedra are called the Platonic solids named after the Greek philosopher and Mathematician, Plato. Each of the Platonic solids can be inscribed inside a sphere as they are considered to be regular 3D polyhedra. In order to count the number of faces, edges and vertices of a 3D shape:. Includes reasoning and applied questions. Faces, edges and vertices is part of our series of lessons to support revision on 3D shapes. You may find it helpful to start with the main 3D shapes lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:. A vertex is a corner where at least 2 edges meet. A triangular prism has 5 faces and 6 vertices. A dodecahedron has 12 faces and 30 edges.

Determine Sides and Vertices Game.

Vertices, Faces and Edges are the three properties that define any three-dimensional solid. A vertex is the corner of the shape whereas a face is a flat surface and an edge is a straight line between two faces. In our day-to-day life activities, we come across a number of objects of different shapes and sizes. There are golf balls, doormats, ice-cream cones, coke cans and so on. These objects have different characteristic properties such as length, breadth, diameter, etc.

Leaning on what makes a solid, identify and count the elements, including faces, edges, and vertices of prisms, cylinders, cones. Gayle chose migratory birds as the topic of her biology class project. She has chosen 12 pictures of different birds to display as part of her photo essay. She plans to purchase cube-shaped photo frames that allow her to place a photo on each side of the cube. How many of the photo frames does Gayle need to frame the 12 pictures? In this concept, you will learn to identify the faces, edges, and vertices of solid figures. A solid figure can be defined by the number and combination of certain parts. These parts are:.

Relationship between faces edges and vertices

Every geometric shape is composed of different parts such as vertices, faces, edges. We come across different objects with rectangular faces, circular faces, cubic faces, diamond faces, triangular faces, etc. We also know many objects that have sharp corners and edges.

Guardian gw2 build

What are Faces, Edges and Vertices? The formal definition for the vertex meaning in Maths is defined as a point where two or more edges meet. Different 3D shapes have different numbers of vertices. According to the theorem, for polyhedrons, the number of faces plus the number of vertices minus the number of edges is equal to 2. It is also important to know that as our reality is constructed in 3 dimensions, it is impossible to physically handle 2d shapes as we are surrounded by 3-dimensional shapes. They are not equal for two-dimensional shapes like circle and oval as they are not polygons. For example, a tetrahedron has 4 faces one of which is not visible. Share your suggestions to enhance the article. Faces, edges and vertices are features of a 3D shape. Different plane shapes have different characteristics, like the number of vertices, the number of sides, etc. A cuboid has 6 rectangular-shaped faces, where the opposite faces are the same.

Three dimensional shapes can be picked up and held because they have length, width and depth.

How many faces, edges, and vertices does a cube have? Correct answer is: 1 A cone has only one curved edge. Faces, Edges And Vertices Here we will learn about faces, edges and vertices including how to calculate the number of vertices, edges and faces of a 3D shape, and how to classify polyhedrons given the number of faces, edges and vertices. The plural of vertex is vertices. Correct Incorrect. Solution: Given, a cube has 6 faces, 12 edges and 8 vertices. The vertical sides of the cylinder are not edges, they just show the curved face of the cylinder. Please read our Cookies Policy for information on how we use cookies and how to manage or change your cookie settings. In order to access this I need to be confident with: Polygons Types of quadrilaterals Triangles 3D shapes Adding and subtracting negative numbers. Help us improve. A line segment between faces is known as an edge.