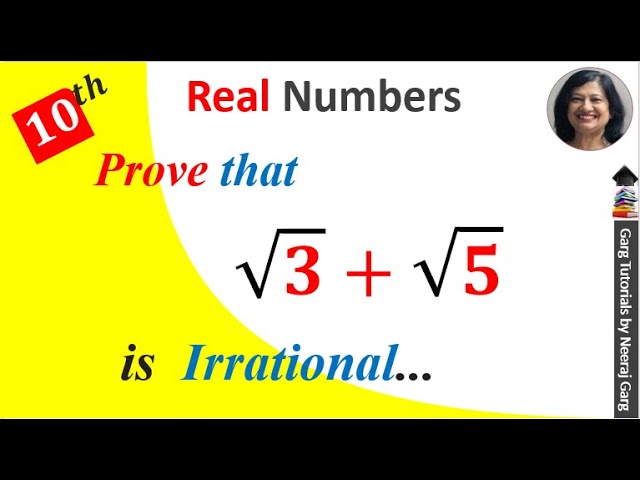
Prove that root 3 and root 5 is irrational

Doc 14 Pages. Doc 16 Pages.

Doc 16 Pages. Doc 7 Pages. Doc 25 Pages. Doc 14 Pages. Doc 18 Pages. Sign in Open App. Most Upvoted Answer.

Prove that root 3 and root 5 is irrational

.

View answer.

.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Proofs concerning irrational numbers. About About this video Transcript. Sal proves that the square root of any prime number must be an irrational number. Created by Sal Khan. Want to join the conversation?

Prove that root 3 and root 5 is irrational

Is root 3 an irrational number? Numbers that can be represented as the ratio of two integers are known as rational numbers, whereas numbers that cannot be represented in the form of a ratio or otherwise, those numbers that could be written as a decimal with non-terminating and non-repeating digits after the decimal point are known as irrational numbers. The square root of 3 is irrational. It cannot be simplified further in its radical form and hence it is considered as a surd. Now let us take a look at the detailed discussion and prove that root 3 is irrational. The square root of a number is the number that when multiplied by itself gives the original number as the product. A rational number is defined as a number that can be expressed in the form of a division of two integers, i.

Grass bagger for zero turn

Prove that Root 3 is irrational.? Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class Forgot Password. Explore all Class 10 Courses. Scan the QR code to for best learning experience! View answer. View All Tests. Doc 18 Pages. Get App. Community Answer. Share with a Friend. The Question and answers have been prepared according to the Class 10 exam syllabus. View answers on App. For Your Perfect Score in Class View all answers.

.

Get App. View courses related to this question Explore Class 10 courses. Join with a free account. Top Courses for Class View all. View all answers and join this discussion on the EduRev App. Signup now for free. Explore Courses. This is a contradiction, so our assumption must be false. Signup for Free! View all answers. Download the App. Doc 18 Pages. Signup now for free. Answer this doubt.