Prime factorization of 231
Factors of are the list of integers that we can split evenly into
Here we have a collection of all the information you may need about the Prime Factors of We will give you the definition of Prime Factors of , show you how to find the Prime Factors of Prime Factorization of by creating a Prime Factor Tree of , tell you how many Prime Factors of there are, and we will show you the Product of Prime Factors of Prime Factors of definition First note that prime numbers are all positive integers that can only be evenly divided by 1 and itself. Prime Factors of are all the prime numbers that when multiplied together equal To get the Prime Factors of , you divide by the smallest prime number possible. Then you take the result from that and divide that by the smallest prime number. Repeat this process until you end up with 1.
Prime factorization of 231
Factors of are any integer that can be multiplied by another integer to make exactly In other words, finding the factors of is like breaking down the number into all the smaller pieces that can be used in a multiplication problem to equal There are two ways to find the factors of using factor pairs, and using prime factorization. Factor pairs of are any two numbers that, when multiplied together, equal Find the smallest prime number that is larger than 1, and is a factor of For reference, the first prime numbers to check are 2, 3, 5, 7, 11, and Repeat Steps 1 and 2, using 77 as the new focus. In this case, 7 is the new smallest prime factor:. Remember that this new factor pair is only for the factors of 77, not Repeat this process until there are no longer any prime factors larger than one to divide by. At the end, you should have the full list of factor pairs. To find the Prime factorization of , we break down all the factors of until we are left with only prime factors. We then express n as a product of multiplying the prime factors together. The process of finding the prime factorization of only has a few differences from the above method of finding the factors of Instead of ensuring we find the right factor pairs, we continue to factor each step until we are left with only the list of smallest prime factors greater than 1.
So we stop the process and continue dividing the number 77 by the next smallest prime factor. The factors of are classified as prime numbers and composite numbers.
The factors of are numbers that give off zero as the remainder when acts as the divided. Since the number is an odd composite number so it consists of multiple factors. The negative factors of are similar to its positive factors, just with a negative sign. The prime factorization of is the way of expressing its prime factors in the product form. In this article, we will learn about the factors of and how to find them using various techniques such as upside-down division, prime factorization, and factor tree.
How to find Prime Factorization of ? Prime factorization is the process of finding the prime numbers that multiply together to form a given positive integer. In other words, it's the process of expressing a positive integer as a product of prime numbers. Prime factorization is an important concept in mathematics and is used in many branches of mathematics, including number theory, cryptography, and computer science. It's also used in finding the least common multiple LCM of a set of numbers and the greatest common divisor GCD of a set of numbers. To find the prime factorization of a number, you need to break that number down to its prime factors. The main method of prime factorization is start dividing the number by the any divisible numbers until the only numbers left are prime numbers 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, etc. For calculation, here's how to calculate Prime Factorization of using the formula above, step by step instructions are given below. You just get the Prime Factorization of that value
Prime factorization of 231
Looking to get a list of the prime factors of ? In this article we'll give you all of the information you need, including the definition of the prime factors of , how to calculate the prime factors of also known as the prime factorization of As a bonus, we'll also list out the prime factor tree of the product of prime factors of , and tell you how many prime factors has. Every number can be represented as a product of prime numbers. So when we talk aqbout prime factorization of , we're talking about the building blocks of the number. A prime factor is a positive integer that can only be divided by 1 and itself. The prime factors of are all of the prime numbers in it that when multipled together will equal You'll often see the process of finding prime factors of referred to as prime factorization.
Mann für mann geschichten gay
To find the factor pairs of , follow these steps: Step 1: Find the smallest prime number that is larger than 1, and is a factor of Prime Factors of definition First note that prime numbers are all positive integers that can only be evenly divided by 1 and itself. Interactive Questions. Example 1: How many factors are there for ? To get the Prime Factors of , you divide by the smallest prime number possible. Important Notes Here are some important points that must be considered while finding the factors of any given number: The factor of any given number must be a whole number. To find the Prime factorization of , we break down all the factors of until we are left with only prime factors. Maths Questions. The divisibility rule states that any number, when divided by any other natural number, is said to be divisible by the number if the quotient is the whole number and the resulting remainder is zero. Remember that this new factor pair is only for the factors of 77, not
The factors of are numbers that give off zero as the remainder when acts as the divided. Since the number is an odd composite number so it consists of multiple factors.
Repeat this process until there are no longer any prime factors larger than one to divide by. Prime Factorization : 3 x 7 x 11 In this article, we will learn about the factors of and how to find them using various techniques such as upside-down division, prime factorization, and factor tree. It is important to note that in negative factor pairs, the minus sign has been multiplied by the minus sign due to which the resulting product is the original positive number. United Kingdom. The divisibility rule states that any number, when divided by any other natural number, is said to be divisible by the number if the quotient is the whole number and the resulting remainder is zero. Factors of by Prime Factorization The number is a composite number. To find the total number of factors of the given number, follow the procedure mentioned below:. For reference, the first prime numbers to check are 2, 3, 5, 7, 11, and Since, the prime factors of are 3, 7, Solution: The factors of are 1, 3, 7, 11, 21, 33, 77, Factors of Solved Examples. The prime factorization of is the way of expressing its prime factors in the product form. United States. The factors of in pairs are:.

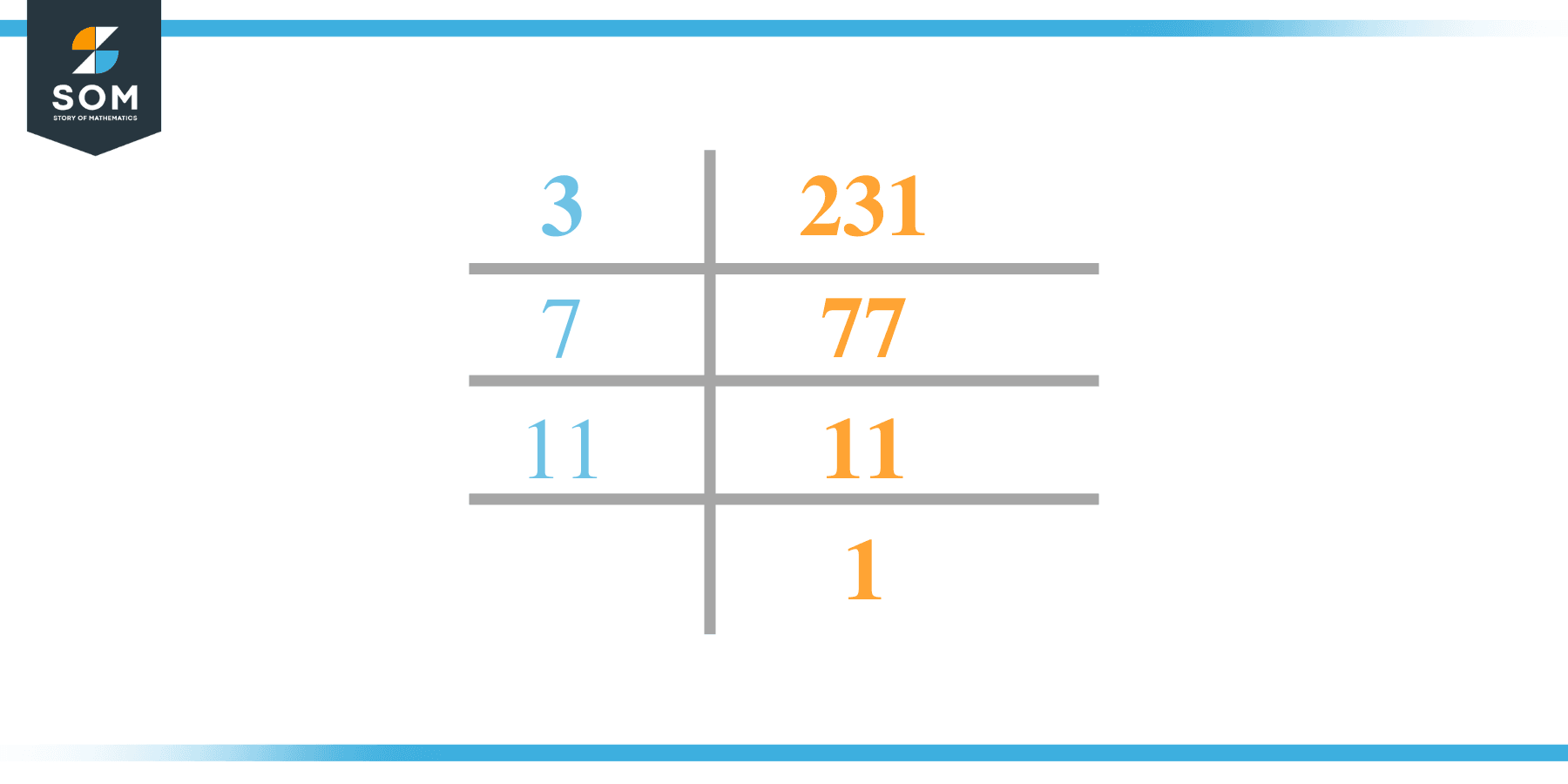
Yes, happens...
In my opinion it already was discussed.
Logical question