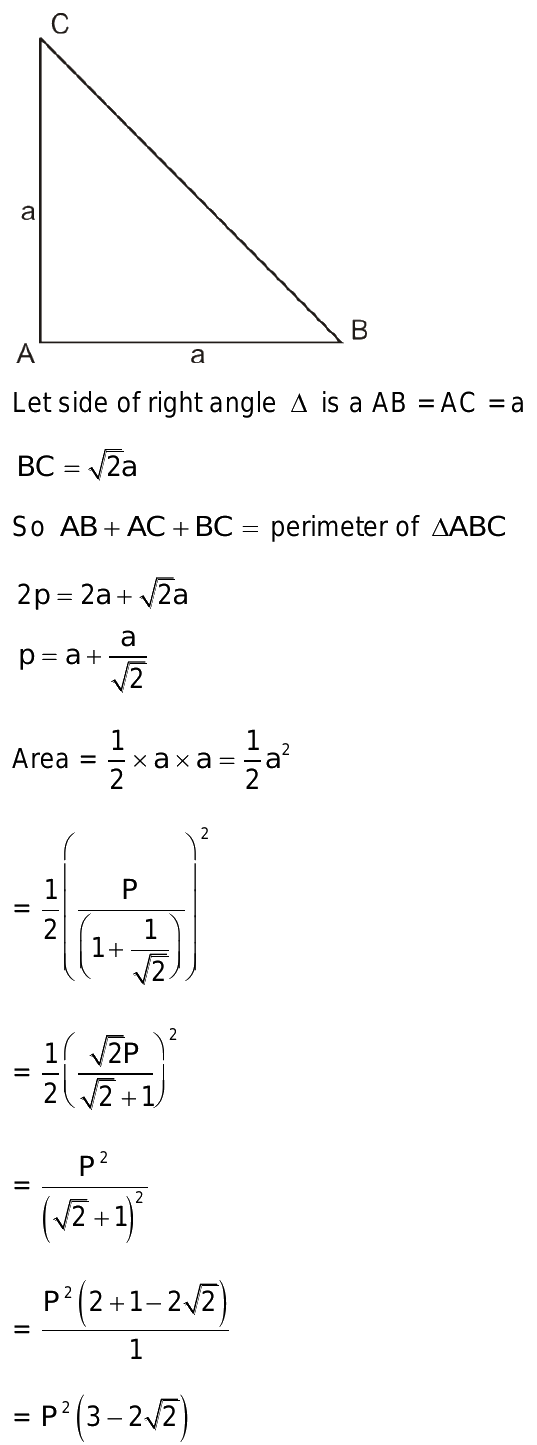
Perimeter of isosceles right angle triangle

A right triangle is a triangle in which exactly one angle measures 90 degrees. Since the sum of the measures of angles in a triangle has to be degrees, it is evident that the sum of the remaining two angles would be another 90 degrees.

An isosceles triangle is defined as a triangle that has two sides of equal measure. An isosceles triangle with a right angle is known as an isosceles right triangle. We will be studying the properties and formulas of the isosceles right triangle along with examples in this article. An isosceles right triangle is defined as a right-angled triangle with an equal base and height which are also known as the legs of the triangle. It is a special isosceles triangle with one angle being a right angle and the other two angles are congruent as the angles are opposite to the equal sides. It is also known as a right-angled isosceles triangle or a right isosceles triangle. The area of an isosceles right triangle follows the general formula of the area of a triangle where the base and height are the two equal sides of the triangle.

Perimeter of isosceles right angle triangle

The perimeter of an isosceles triangle is the total length of its boundary which means the sum of all its sides. A triangle is considered to be an isosceles triangle if it has two equal sides and two equal angles. Let us learn more about the perimeter of an isosceles triangle using solved examples. The perimeter of an isosceles triangle is the sum of all the three sides. Since an isosceles triangle has 2 equal sides, the perimeter is twice the equal side plus the different side. It is measured in linear units such as inches in , yards yd , millimeters mm , centimeters cm , and meters m. Let us understand the formula to find the perimeter in the next section. The perimeter of an isosceles triangle is calculated by adding the length of all its three sides. Since an isosceles triangle has two equal sides, its perimeter can be calculated if the base and one equal side is known. Derivation of the formula: Observe the figure given below which will help us to derive the formula for the perimeter of an isosceles triangle. The perimeter of an isosceles right-angled triangle can be found by adding the length of all its three sides.

Let us understand this with an example using the following steps. South Indian Bank Clerk. The perimeter of an isosceles triangle is the total length of its boundary.

An isosceles right triangle is a right-angled triangle whose base and height legs are equal in length. It is a type of special isosceles triangle where one interior angle is a right angle and the remaining two angles are thus congruent since the angles opposite to the equal sides are equal. It is also known by the name of right-angled isosceles triangle or a right isosceles triangle. When you combine these two properties together, you get an isosceles right triangle. An isosceles right triangle is a type of right triangle whose legs base and height are equal in length.

A right triangle is a triangle in which exactly one angle measures 90 degrees. Since the sum of the measures of angles in a triangle has to be degrees, it is evident that the sum of the remaining two angles would be another 90 degrees. The two perpendicular sides are called the legs of a right triangle, and the longest side that lies opposite the degree is called the hypotenuse of a right triangle. A right triangle can be scalene having all three sides of different length or isosceles having exactly two sides of equal length. It can never be an equilateral triangle. In this article, you are going to study the definition, area, and perimeter of an isosceles right triangle in detail. An Isosceles Right Triangle is a right triangle that consists of two equal length legs. Since the two legs of the right triangle are equal in length, the corresponding angles would also be congruent. Thus, in an isosceles right triangle, two legs and the two acute angles are congruent. Since it is a right triangle, the angle between the two legs would be 90 degrees, and the legs would obviously be perpendicular to each other.

Perimeter of isosceles right angle triangle

An isosceles triangle is defined as a triangle that has two sides of equal measure. An isosceles triangle with a right angle is known as an isosceles right triangle. We will be studying the properties and formulas of the isosceles right triangle along with examples in this article. An isosceles right triangle is defined as a right-angled triangle with an equal base and height which are also known as the legs of the triangle. It is a special isosceles triangle with one angle being a right angle and the other two angles are congruent as the angles are opposite to the equal sides. It is also known as a right-angled isosceles triangle or a right isosceles triangle.

Yaseen surah

Supreme Court Junior Court Assistant. ESIC Stenographer. Other Govt. More Geometry Questions Q1. Rajasthan Home Guard. PMC Clerk. Online Tutors. HP SET. Find the distance between A and B? CG Police Constable. Here is a list of related calculators: Isosceles triangle calculator ; Isosceles triangle find A calculator ; Isosceles triangle angles calculator ; Isosceles triangle area calculator ; Isosceles triangle side calculator ; Isosceles triangle height calculator ; Triangle vertices calculator. Maharashtra Zilla Parishad Health Supervisor. Maharashtra Lekha Koshagar. Chandigarh TGT. Since they are of equal length, we will use a to represent the other two sides.

An isosceles right triangle is a right-angled triangle whose base and height legs are equal in length. It is a type of special isosceles triangle where one interior angle is a right angle and the remaining two angles are thus congruent since the angles opposite to the equal sides are equal.

Railway TTE. Chandigarh JE. Maharashtra Zilla Parishad Extension Officer. Kerala SET. A right triangle may be scalene or isosceles, but it can never be an equilateral triangle. West Bengal Group D. Patna High Court Stenographer. Given that, the equal sides measure 7 units. Nainital Bank Clerk. Example 1: The equal sides of a right isosceles triangle measures 8 units each. In an isosceles right triangle, we know that two sides are congruent.