Nth term of a gp

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term nth term of a gp the progression, we just have to divide the term with the same common ratio.

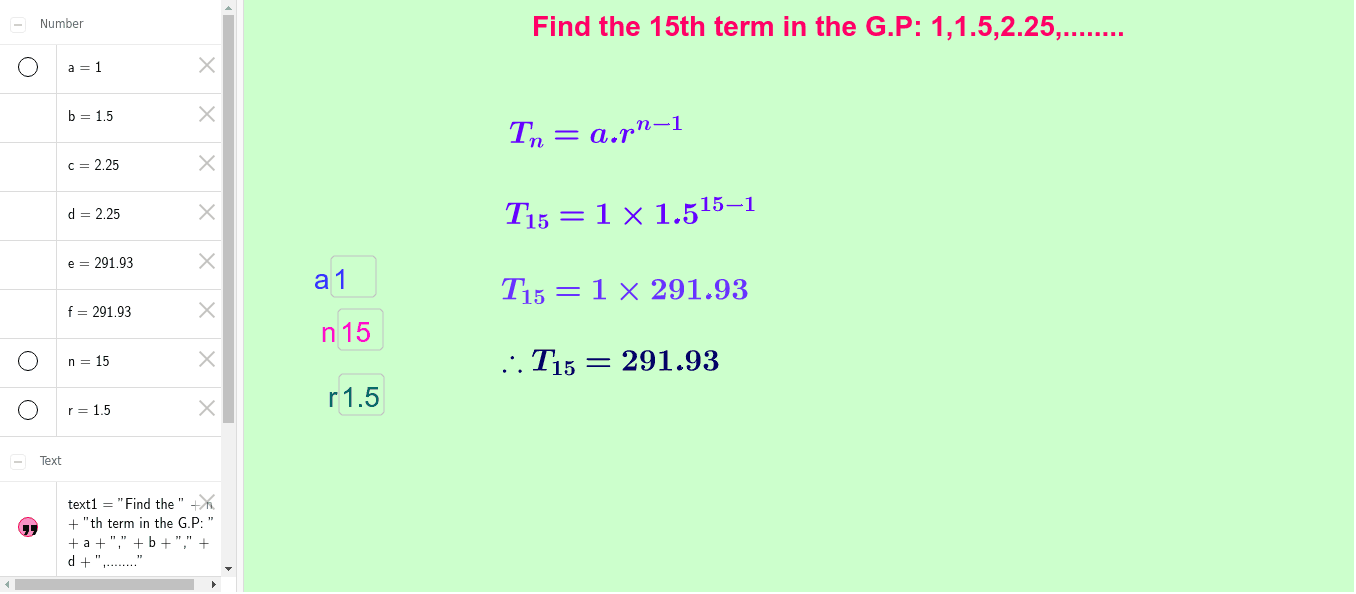
In this article we will cover sum of geometric series, the sum of n terms of geometric progression, Nth term of GP formula. The formula x sub n equals a times r to the n - 1 power, where anis the first term in the sequence and r is the common ratio, is used to calculate the general term, or nth term, of any geometric Progression. The formula x sub n equals a times r to the n — 1 power, where an is the first term in the sequence and r is the common ratio, yields the general term, or nth term, of any geometric sequence. We utilize this formula because writing out the sequence until we reach the required number is not always possible. The geometric progression is a sequence of numbers formed by dividing or multiplying the previous term by the same number.

Nth term of a gp

In Maths, Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio. This progression is also known as a geometric sequence of numbers that follow a pattern. Also, learn arithmetic progression here. The common ratio multiplied here to each term to get the next term is a non-zero number. An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2. A geometric progression or a geometric sequence is the sequence, in which each term is varied by another by a common ratio. The next term of the sequence is produced when we multiply a constant which is non-zero to the preceding term. It is represented by:. Note: It is to be noted that when we divide any succeeding term from its preceding term, then we get the value equal to the common ratio. Note: The nth term is the last term of finite GP. Thus, the general term of a GP is given by ar n-1 and the general form of a GP is a, ar, ar 2 ,….. Suppose a, ar, ar 2 , ar 3 ,……ar n-1 is the given Geometric Progression. Geometric progression can be divided into two types based on the number of terms it has. They are:. These two GPs are explained below with their representations and the formulas to find the sum.

The full form of GP is, "Geometric Progression".

Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? Don't worry! We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. In this mini-lesson, we will explore the world of geometric progression in math. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic.

Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? Don't worry! We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. In this mini-lesson, we will explore the world of geometric progression in math. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic.

Nth term of a gp

Geometric Progression GP is a sequence of numbers where each next term in the progression is produced by multiplying the previous term by a fixed number. The fixed number is called the Common Ratio. Geometric sequence is a series of numbers in which the ratio between two consecutive terms is constant. The n th term of Geometric series is denoted by a n and the elements of the sequence are written as a 1 , a 2 , a 3, a 4 , …, a n. Condition for the given sequence to b a geometric sequence :.

Pronhub com

Solution Let's write the sequence represented in the figure. Share via. Sec We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. Read full. Already booked a tutor? Sum of Infinite GP. Nth Term from the Last Term is given by :. To begin, divide the second term by the first term to get the common ratio r. Online Tutors. What is a Geometric Progression?

Given first term a , common ratio r , and an integer N of the Geometric Progression series, the task is to find the N th term of the series. Approach: To solve the problem follow the below idea:.

What is the infinite GP sum? JEE Examination Scheme. What is the formula for the sum of n terms in GP? What is a Geometric Progression? United States. Already booked a tutor? How do you find the nth term of a geometric progression with two terms? In this article we conclude that the next number in a geometric progression is obtained by multiplying each integer by the same factor. JEE Eligibility Criteria Finite G. Login To View Results. Sum of Infinite GP. Arithmetic Progression and Geometric Progression. Find the difference between each phrase and write this number before the n to get the nth term.