Moment of inertia of a sphere
The moment of inertia of a sphere is a significant concept in physics. It's often represented as follows:. In this equation, R signifies the radius of the sphere and M represents its mass. The formula above specifically pertains to a solid sphere's moment of inertia about its central axis.
Moment of inertia , denoted by I , measures the extent to which an object resists rotational acceleration about a particular axis , it is the rotational analogue to mass which determines an object's resistance to linear acceleration. It should not be confused with the second moment of area , which has units of dimension L 4 [length] 4 and is used in beam calculations. The mass moment of inertia is often also known as the rotational inertia , and sometimes as the angular mass. For simple objects with geometric symmetry, one can often determine the moment of inertia in an exact closed-form expression. Typically this occurs when the mass density is constant, but in some cases the density can vary throughout the object as well. In general, it may not be straightforward to symbolically express the moment of inertia of shapes with more complicated mass distributions and lacking symmetry.
Moment of inertia of a sphere
.
Secondly, we can sum up the moments of these exceedingly small thin disks along a given axis.
.
In the preceding subsection, we defined the moment of inertia but did not show how to calculate it. In this subsection, we show how to calculate the moment of inertia for several standard types of objects, as well as how to use known moments of inertia to find the moment of inertia for a shifted axis or for a compound object. This section is very useful for seeing how to apply a general equation to complex objects a skill that is critical for more advanced physics and engineering courses. In this case, the summation over the masses is simple because the two masses at the end of the barbell can be approximated as point masses, and the sum therefore has only two terms. In the case with the axis at the end of the barbell—passing through one of the masses—the moment of inertia is. From this result, we can conclude that it is twice as hard to rotate the barbell about the end than about its center. In this example, we had two point masses and the sum was simple to calculate. However, to deal with objects that are not point-like, we need to think carefully about each of the terms in the equation. Recall that in our derivation of this equation, each piece of mass had the same magnitude of velocity, which means the whole piece had to have a single distance r to the axis of rotation.
Moment of inertia of a sphere
In this article, we will learn the moment of inertia of Solid or Hollow Sphere, along with examples, calculation, etc. The moment of inertia is also known as the second moment of the area, and it can be calculated for various objects having different shapes. The moment of inertia of a sphere is defined as the summation of the products from the whole mass of every attached element of the entity and then multiplying them by the square of the particles with reference to its distance from the center. There is one formula to calculate the moment of inertia of a solid sphere also known as a spherical shell.
Challenger breaker box
The Mathematical Gazette. The formula above specifically pertains to a solid sphere's moment of inertia about its central axis. This list of moment of inertia tensors is given for principal axes of each object. Plane polygon with vertices P 1 , P 2 , P 3 , Following are scalar moments of inertia. The next step involves adding x into the equation. Considering the diagram, we can see that r, R, and x form a triangle. Moment of inertia , denoted by I , measures the extent to which an object resists rotational acceleration about a particular axis , it is the rotational analogue to mass which determines an object's resistance to linear acceleration. The mass moment of inertia is often also known as the rotational inertia , and sometimes as the angular mass. In the above table, n would be the unit Cartesian basis e x , e y , e z to obtain I x , I y , I z respectively. This expression assumes that the rod is an infinitely thin but rigid wire.
Moment of inertia , denoted by I , measures the extent to which an object resists rotational acceleration about a particular axis , it is the rotational analogue to mass which determines an object's resistance to linear acceleration. It should not be confused with the second moment of area , which has units of dimension L 4 [length] 4 and is used in beam calculations.
It's often represented as follows:. Contents move to sidebar hide. Download as PDF Printable version. Download as PDF. Regular dodecahedron of side s and mass m. Plane regular polygon with n -vertices and mass m uniformly distributed on its interior, rotating about an axis perpendicular to the plane and passing through its barycenter. Deriving the Moment of Inertia of a Sphere We can derive the moment of inertia of a sphere in two primary ways: Firstly, we can slice the solid sphere into infinitesimally thin solid cylinders. Thin rod of length L and mass m , perpendicular to the axis of rotation, rotating about its center. Thin rod of length L and mass m , perpendicular to the axis of rotation, rotating about one end. Ellipsoid solid of semiaxes a , b , and c with mass m. For simple objects with geometric symmetry, one can often determine the moment of inertia in an exact closed-form expression. Tools Tools. Wolfram Research. Report An Error.

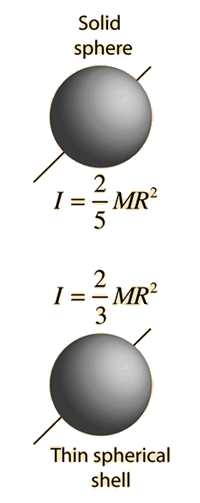
0 thoughts on “Moment of inertia of a sphere”