Khan academy finite element method

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos.

Personalise your OpenLearn profile, save your favourite content and get recognition for your learning. Start this free course now. Just create an account and sign in. Enrol and complete the course for a free statement of participation or digital badge if available. The basic principles underlying the FEM are relatively simple. Consider a body or engineering component through which the distribution of a field variable, e. Examples could be a component under load, temperatures subject to a heat input, etc.

Khan academy finite element method

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Modeling situations with differential equations. About About this video Transcript. Differential equations are equations that relate a function with one or more of its derivatives. This means their solution is a function! Learn more in this video. Want to join the conversation? Log in. Sort by: Top Voted. Posted 9 years ago. At Downvote Button navigates to signup page. Flag Button navigates to signup page.

By power rule, it is 2x. Search for courses, skills, and videos.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Linear algebra. Unit 1.

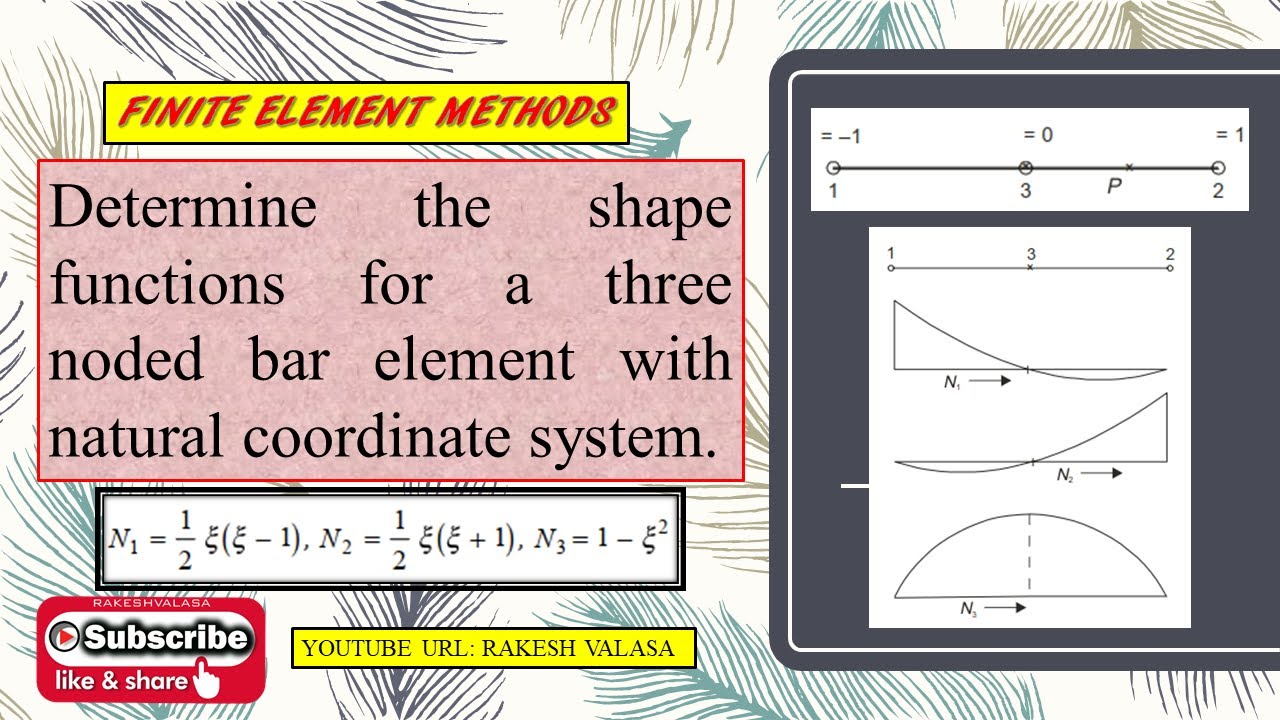
The finite element method FEM is a powerful technique originally developed for numerical solution of complex problems in structural mechanics , and it remains the method of choice for complex systems. In the FEM, the structural system is modeled by a set of appropriate finite elements interconnected at discrete points called nodes. Elements may have physical properties such as thickness, coefficient of thermal expansion , density , Young's modulus , shear modulus and Poisson's ratio. The origin of finite method can be traced to the matrix analysis of structures [1] [2] where the concept of a displacement or stiffness matrix approach was introduced. Finite element concepts were developed based on engineering methods in s.

Khan academy finite element method

Forgot password? New user? Sign up. Existing user? Log in. Already have an account?

Heritage rbc leaderboard

This may seem to be a bit of a liberty, but it can be surprisingly close to reality. Search for courses, skills, and videos. As Sal mentioned at Orthogonal complements : Alternate coordinate systems bases Orthogonal projections : Alternate coordinate systems bases Change of basis : Alternate coordinate systems bases. Forever Learner. So that's going to be two times this right over here. The first step, known as the base case, is to prove the given statement for the first natural number. All of that over 2. Show preview Show formatting options Post answer. Posted 10 years ago. The elements are assumed to be connected to one another, but only at interconnected joints, known as nodes. Video transcript - [Voiceover] Let's now introduce ourselves to the idea of a differential equation.

If you're seeing this message, it means we're having trouble loading external resources on our website.

Mathematical induction is a method of mathematical proof typically used to establish a given statement for all natural numbers. Doing a ton of practice problems really really helps. We apologise for the inconvenience. The notation is just sloppy. So you would know what I'm doing. And we are done. And so the solutions, or the solution, is going to be a function or a set of functions. Your statement might be true for everything above It is important to note that the elements are notionally small regions, not separate entities like bricks, and there are no cracks or surfaces between them. And the reason why this works is - Let's say that we prove both of these. So it definitely will work for 2. So we have nine e to the negative three x plus two times the first derivative.