Formula of inscribed angle
Note: The term "intercepted arc" refers to an arc "cut off" or "lying between" the sides of the specified angle.
A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. The only way to gather all the points that are the same distance from a point is to create a curved line. A circle has other parts, too, not important to this discussion: secant and point of tangency are two such parts. Circles are almost always indicated by the mathematical symbol followed by the circle's letter designation, its center point. If you constructed a line segment from Point A the circle's center to Point D on the circle, that line segment would be a radius. Running a chord from Point B to Point E would give you a diameter, which must run through the center of the circle. With circles, geometry becomes at once more interesting and more difficult.
Formula of inscribed angle
Welcome to our inscribed angle calculator , the perfect tool for calculating the angle inscribed by two chords in a circle. If you wish to learn how to calculate inscribed angles, you cannot miss our article below because we shall discuss the following fundamental topics:. The inscribed angle theorem establishes a relationship between the central and inscribed angles. It states that:. We now know how to calculate the inscribed angle from its central angle. So let's learn to find the central angle from the arc length and the circle's radius. Are you intrigued to learn more about the arc length? Head to our arc length calculator! If you're burning with the question how to calculate the arc length with an inscribed angle , we shall combine the above relation with the inscribed angle formula to obtain the following:. Enter the central angle , and our calculator will find the inscribed angle for you.
But when P is in the minor arc shortest arc between A and Bthe angle is still constant, formula of inscribed angle, but is the supplement of the usual measure. A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. Inscribed Angle in a Semicircle, StudySmarter Originals Formula of inscribed angle Q uadrilateral If a quadrilateral is inscribed in a circle, which means that the quadrilateral is formed in a circle by chords, then its opposite angles are supplementary.
Definition: An inscribed angle is an angle whose vertex lies on the circumference of the circle. The vertex is the common endpoint of the two sides of the angle. An inscribed angle can be defined as the angle subtended at a point on the circle by two given points on the circle. An inscribed angle is an angle formed in the interior of a circle by two chords that have a common endpoint on the circle. The inscribed angle is used in many proofs of elementary Euclidean geometry of the plane. Inscribed angle is the basis for several other theorems related to the power of a point with respect to a circle.
Home » Geometry » Angle » Inscribed Angle. An inscribed angle is an angle whose vertex lies on the circumference of a circle while its two sides are chords of the same circle. The arc formed by the inscribed angle is called the intercepted arc. In order to prove this theorem, we need to consider three separate cases. Each of them will differ based on where the center lies in comparison to the inscribed angle. Case 1: Prove Inscribed Angle Theorem when the inscribed angle is between a chord and the diameter of a circle.
Formula of inscribed angle
The circular geometry is really vast. A circle consists of many parts and angles. These parts and angles are mutually supported by certain Theorems, e. Circles are all around us in our world. There exists an interesting relationship among the angles of a circle. Three types of angles are formed inside a circle when two chords meet at a common point known as a vertex. These angles are the central angle, intercepted arc, and the inscribed angle. An inscribed angle is an angle whose vertex lies on a circle, and its two sides are chords of the same circle.
Food basics flyer
The only way to gather all the points that are the same distance from a point is to create a curved line. Start Quiz. Combining these results with equation 2 yields. If the point is in the minor arc, then the will produce the supplement of the correct result, but the the length of the minor arc should still be used in the formula. Please read the " Terms of Use ". If you're burning with the question how to calculate the arc length with an inscribed angle , we shall combine the above relation with the inscribed angle formula to obtain the following:. When two chords intersect inside a circle, four angles are formed. The intercepted arcs are arc and arc. Learn with 2 Inscribed Angles flashcards in the free StudySmarter app. Create your free account now. Central Angle A central angle is an angle formed by two radii with the vertex at the center of the circle. Angle bisector theorem Exterior angle theorem Euclidean algorithm Euclid's theorem Geometric mean theorem Greek geometric algebra Hinge theorem Inscribed angle theorem Intercept theorem Intersecting chords theorem Intersecting secants theorem Law of cosines Pons asinorum Pythagorean theorem Tangent-secant theorem Thales's theorem Theorem of the gnomon.
An inscribed angle is an angle whose vertex is on a circle and whose sides contain chords of a circle. This is different than the central angle, whose vertex is at the center of a circle.
An inscribed angle is an angle whose vertex lies on a circle and its two sides are chords of the same circle. In a circle, or congruent circles, congruent central angles have congruent arcs. Join over 22 million students in learning with our StudySmarter App. What is the formula for calculating inscribed angles? Angle Formed by Two Intersecting Chords. Combining these results with equation 2 yields. Refer to the above figure. Try this Drag any orange dot. An arc of a circle is a curve formed by two points in a circle. Circumference to diameter. Statistics Tutors near me. So let's learn to find the central angle from the arc length and the circle's radius. Calculus Tutors near me.

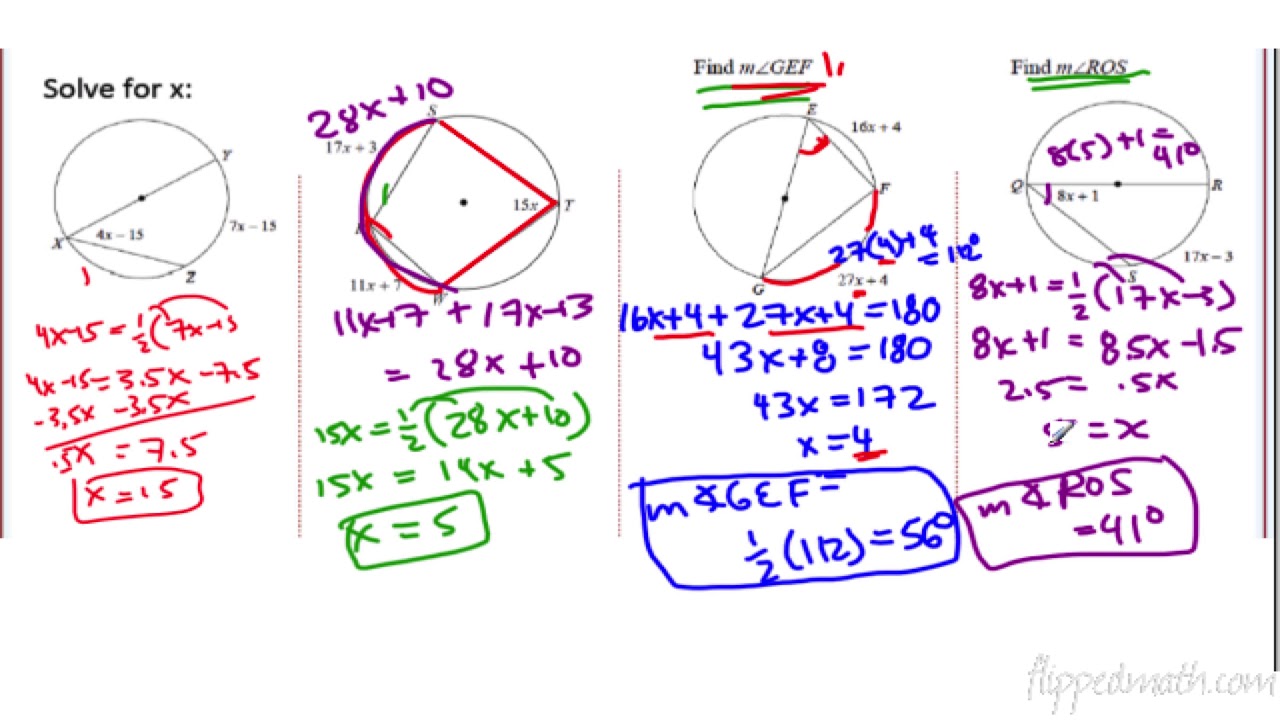
0 thoughts on “Formula of inscribed angle”