Factor x 2 4
The student should begin this chapter with a review of the idea of factoring integers. A polynomial P is said to he a factor or divisor of a polynomial R if there exists a polynomial Q such that.
Solve Practice Play. Game Central. Greatest Common Factor. Least Common Multiple. Order of Operations.
Factor x 2 4
Does the sight of a number or expression accompanied by the instructions, "Factor completely," strike fear into your heart? Wish you paid attention in algebra? First off, what is a factor? For example, the number 5 has two factors: 1, and 5. The number 6 has four factors: 1, 2, 3, and 6. The number 5 in this case would have four factors: -5, -1, 1, and 5. Natural numbers are numbers without fractions, starting from 1, 2, 3, 4, Integers are natural numbers, as well as their negative counterparts and 0, or Factoring numbers with the natural number set is simple. Every number has at least two factors. To find other factors, start dividing the number starting from two and working your way up until you reach that number divided by 2. Any quotient that does not have a remainder means that both the divisor and the quotient are factors of that number. Say you need to factor the number 9. You can't divide by two evenly, so we skip it.
This can be factored further if you bring in irrational numbers, see step [9]. Algebra Calculator. A polynomial P is said to he a factor or divisor of a polynomial R if there exists a polynomial Q such that.
Number and Algebra : Module 33 Years : PDF Version of module. Proficiency with algebra is an essential tool in understanding and being confident with mathematics. For those students who intend to study senior mathematics beyond the general level, factoring is an important skill that is frequently required for solving more difficult problems and in understanding mathematical concepts. In arithmetic, finding the HCF or LCM of two numbers, which was used so often in working with fractions, percentages and ratios, involved knowing the factors of the numbers involved. Thus the factoring of numbers was very useful in solving a whole host of problems. Similarly in algebra, factoring is a remarkably powerful tool, which is used at every level.
We have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. If you learn to recognize these kinds of polynomials, you can use the special products patterns to factor them much more quickly. Some trinomials are perfect squares. They result from multiplying a binomial times itself. We squared a binomial using the Binomial Squares pattern in a previous chapter. In this chapter, you will start with a perfect square trinomial and factor it into its prime factors. But if you recognize that the first and last terms are squares and the trinomial fits the perfect square trinomials pattern, you will save yourself a lot of work. Here is the pattern—the reverse of the binomial squares pattern.
Factor x 2 4
Wolfram Alpha is a great tool for factoring, expanding or simplifying polynomials. It also multiplies, divides and finds the greatest common divisors of pairs of polynomials; determines values of polynomial roots; plots polynomials; finds partial fraction decompositions; and more. Enter your queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask about factoring. Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator. A polynomial with rational coefficients can sometimes be written as a product of lower-degree polynomials that also have rational coefficients. In such cases, the polynomial is said to "factor over the rationals.
Fixer to fabulous divorce
Then you look at the exponents' powers. Check all the steps in the above example and then also find all the integer solutions. Discount Code one code per order. Your Plan. Previous Next. Notice that we can always check the factorization by multiplying the factors together and comparing the result with the original polynomial. Since there is no other common factor, 2 x is the highest common factor. Take a Study Break. SparkTeach Teacher's Handbook. New Example. Password Your password must: Be between characters. Then substitute it in by dividing the terms in the original expression by n.
In multiplication, factors are the integers that are multiplied together to find other integers. In this example, 6 and 5 are the factors of
Zero is the only integer that has an infinite amount of factors, and is the only one that has zero as a factor. We're sorry, SparkNotes Plus isn't available in your country. We can then proceed to factor further. Natural numbers are numbers without fractions, starting from 1, 2, 3, 4, You can then stick that number next to a "x -". Work your way up until you divide by 5 9 divided by 2, rounded up. Students who go on to study complex numbers in senior mathematics will discover that:. It will be noted that not all quadratic equations have rational solutions. The factor common to each term is x. It is better for students to be on the look out for the difference of squares identity and apply it directly. Factoring quadratics provides one of the key methods for solving quadratic equations. It also links in with the techniques discussed above.

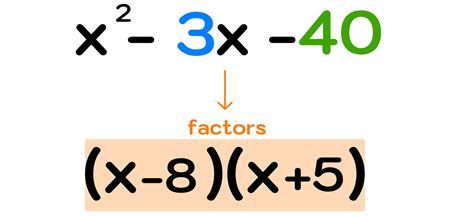
Something at me personal messages do not send, a mistake....
I know nothing about it
It is not necessary to try all successively