Domain and range in a parabola

There are four different common relationships between variables you're sure to run into: they're linear, direct, quadratic, and inverse relationships.

Given a situation that can be modeled by a quadratic function or the graph of a quadratic function, determine the domain and range of the function. A 6 Quadratic functions and equations. The student applies the mathematical process standards when using properties of quadratic functions to write and represent in multiple ways, with and without technology, quadratic equations. The student is expected to:. A 6 A determine the domain and range of quadratic functions and represent the domain and range using inequalities. How do you determine the domain and range of a quadratic function when given a verbal statement? We're going to explore different representations of quadratic functions, including graphs, verbal descriptions, and tables.

Domain and range in a parabola

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Determining the range of a function Algebra 2 level. About About this video Transcript. Want to join the conversation? Log in. Sort by: Top Voted. Posted 11 years ago. Downvote Button navigates to signup page. Flag Button navigates to signup page. Show preview Show formatting options Post answer. Sal mentions how to find the vertex at Comment Button navigates to signup page. Should I take the Quadratics course before Functions?

Introduction There are four different common relationships between variables you're sure to run into: they're linear, direct, quadratic, and inverse relationships. The maximum value is given by.

The domain and range of a function are integral to its definition. In this section, you will learn how to use algebraic techniques to define a function's domain and range given its equation. The general form of a quadratic function presents the function in the form where , and are real numbers and. If , the parabola opens upward. If , the parabola opens downward. We can use the general form of a parabola to find the equation for the axis of symmetry.

The cross-section of the antenna is in the shape of a parabola, which can be described by a quadratic function. In this section, we will investigate quadratic functions, which frequently model problems involving area and projectile motion. Working with quadratic functions can be less complex than working with higher degree functions, so they provide a good opportunity for a detailed study of function behavior. The graph of a quadratic function is a U-shaped curve called a parabola. One important feature of the graph is that it has an extreme point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value.

Domain and range in a parabola

Given a situation that can be modeled by a quadratic function or the graph of a quadratic function, determine the domain and range of the function. A 6 Quadratic functions and equations. The student applies the mathematical process standards when using properties of quadratic functions to write and represent in multiple ways, with and without technology, quadratic equations. The student is expected to:. A 6 A determine the domain and range of quadratic functions and represent the domain and range using inequalities.

Head i sl worldcup rebels

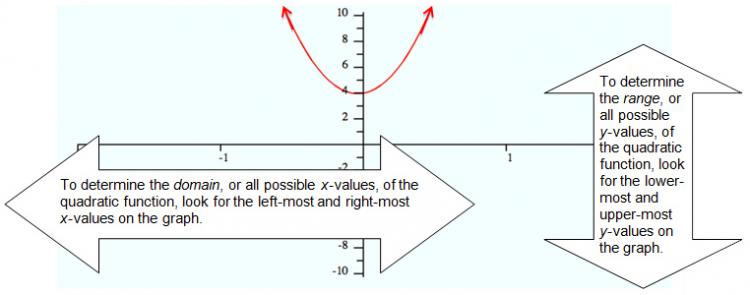
When asked to identify the true statement regarding the independent and dependent variable, choose A, B, or C. Example 4 Find the domain and range of the quadratic function Solution This is also a parabola since quadratic function. Or, we should go from negative 5 all the way to positive 7. The maximum value is given by. Negative b over 2a is the formula for it. Investigating Domain and Range Using Graphs We're going to explore different representations of quadratic functions, including graphs, verbal descriptions, and tables. To determine the domain and range of any function on a graph, the general idea is to assume that they are both real numbers, then look for places where no values exist. Find the vertex of the quadratic function. So, let's say this is negative 1,2,3,4,5. Read on to know more about Record the example problem and the table of values for t and h.

There are four different common relationships between variables you're sure to run into: they're linear, direct, quadratic, and inverse relationships.

In fact, the domain of all quadratic functions is all real numbers! Here are some examples on domain and range of a parabola. Mae Scully. Sometimes you can use a graphing calculator to possess an accurate picture of the function. And, to get a flavor for this, I'm going to try to graph this function right over here. Or, we should go from negative 5 all the way to positive 7. Study Guide. The actual computations I won't cover here, but let us just say that the vertex of a parabola is the only point of the parabola where the ever-changing slope equals zero. See Table 1. But the parabola has a vertex which is a minimum y-value for any parabola that faces up or a maximum y-value for any parabola that faces down.