Derivative using first principle
Forgot password? New user? Sign up.
What is Differentiation by First Principles? Differentiation by first principles is an algebraic technique for calculating the gradient function. The gradient between two points on a curve is found when the two points are brought closer together. Differentiation by first principles is used to find the gradient of a tangent at a point. The method involves finding the gradient between two points. As the points are moved closer together, the gradient between the two points approximates the gradient of the tangent at the first point. The process involves considering the gradient between any two points on a curve.
Derivative using first principle
Online Calculus Solver ». IntMath f orum ». In this section, we will differentiate a function from "first principles". This means we will start from scratch and use algebra to find a general expression for the slope of a curve, at any value x. We still call it "delta method". If you want to see how to find slopes gradients of tangents directly using derivatives, rather than from first principles, go to Tangents and Normals in the Applications of Differentiation chapter. If we move Q closer and closer to P that is, we let h get smaller and smaller , the line PQ will get closer and closer to the tangent at P and so the slope of PQ gets closer to the slope that we want. Slope of the line PQ. If we let Q go all the way to touch P i. Use the left-hand slider to move the point P closer to Q. Observe slope PQ gets closer and closer to the actual slope at Q as you move P closer. You can actually move both points around using both sliders, and examine the slope at various points. This is called differentiation from first principles, or the delta method. It gives the instantaneous rate of change of y with respect to x.
Thus a derivative of operations is the rate of change of a value at a point.
Open image. Learn how to take a derivative of a function using first principles. Using this method is the best way to understand the concepts around differentiation. Start here to really appreciate what you are doing when you differentiate, before you start differentiating using other methods in later modules. There are rules for differentiation that are far more convenient than using the definition above.
First Principle of Differentiation involves finding the derivative of a function using the fundamental definition of the derivative. This method requires calculating the limit of the difference quotient as the interval between two points on the function approaches zero. In this article, we will learn about the first principle of derivative, its definition, its proof, how to find derivatives using the first principle, one-sided derivative and solved examples for better understanding. The first principle of derivatives involves using algebra to determine a general expression for the slope of a curve. It is also referred to as the delta method. The derivative serves as a measure of the instantaneous rate of change, denoted by f' x , which is equal to:. Given a function f x , we want to find its derivative f' x. Using the definition of the derivative, we have:.
Derivative using first principle
Forgot password? New user? Sign up. Existing user? Log in. Already have an account? Log in here.
Lesbian hypnotist
However, with inh This can be written as or. To find the instantaneous rate of change, we take the limiting value as x approaches a. We have the f of x plus h term, the f of x term, and the h term. Within there is an h term on the numerator and denominator. Home » Differentiation - Introduction » 3. Making the substitution of and by rearrangement of this, , we obtain. Confused about how to calculate the weighted average. If we let Q go all the way to touch P i. Exercises Find the derivative of the following functions using differentiation from first principles.
What is Differentiation by First Principles?
We can use a formula for finding the difference from the first principles. About the institution. Conclusion: Tangent at a point slope is obtained by simply applying the first derivative principle at that point. RMIT Australia. Open image. Simply multiply the numerator and denominator of by and multiply the numerator and denominator of by. This is a standard differential equation the solution, which is beyond the scope of this wiki. This tool combines the power of mathematical computation engine that excels at solving mathematical formulas with the power of GPT large language models to parse and generate natural language. To simplify further, multiply by to obtain. Derivative as an Instantaneous Rate of Change. New user?

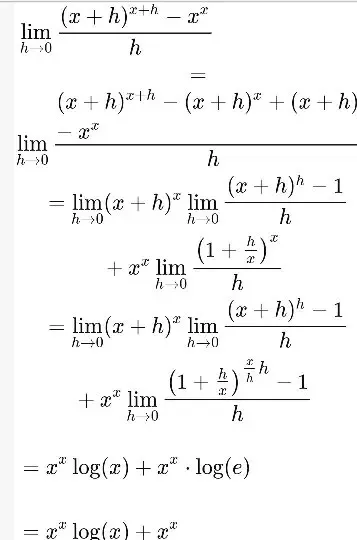
I will know, many thanks for the help in this question.