Decimal expansion of a rational number is terminating

Terminating decimals are the numbers that have a fixed or a finite number of digits after the decimal point. Decimal numbers are used to represent the partial amount of whole, just like fractions. In this lesson, we will focus on the type of decimal numbers, that is, terminating decimal numbers. The word 'terminate' means to bring to an end.

Let's first define rational numbers before discussing how they are represented when expanded to decimal form. Decimals are what is produced when these numbers are further simplified. The decimal expansion of a rational number is obtained by dividing the numerator by the denominator of the rational number. Decimal Expansion. By dividing a rational number's numerator by its denominator, one can get the decimal expansion of a rational number.

Decimal expansion of a rational number is terminating

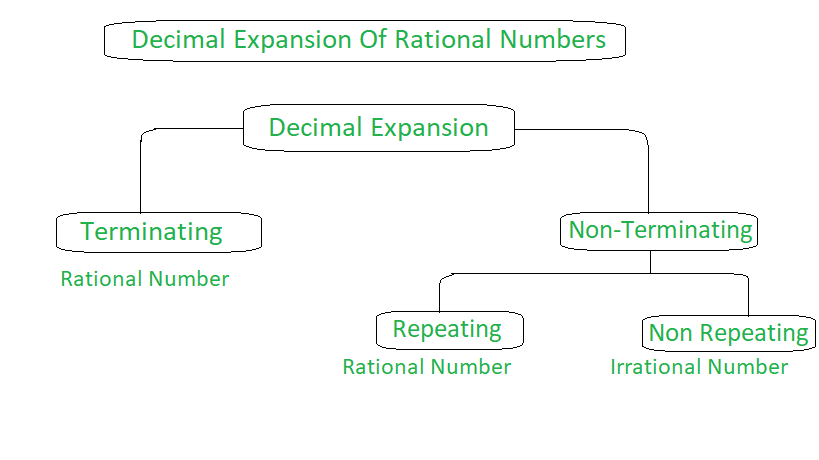
Decimal expansion of rational numbers means when we represent a rational number given in the form of a fraction in the form of a decimal. We use the long division method for this process of conversion. In this math article we will study the decimal expansion of rational numbers. We use the long division method for this process. When we perform the long division method, the quotient obtained after division is the required equivalent value of our rational number in the decimal form. Here as we are not getting the remainder 0 so we terminate the division and consider the quotient got so far. We see that for some rational numbers we get a certain fixed value of the decimal equivalent. But there are also some rational numbers where the division process does not get terminated as shown in the above example. Depending on the remainder we get and the length of the quotient, we can classify the decimal expansion into two types. A terminating decimal expansion of a rational number means that while using the long division method we will get a fixed number of digits in the quotient. In other words, while dividing the remainder becomes zero at some point of time and thus the division process gets terminated. It means that the denominator of the rational number does not have any factors other than 2 and 5. Here we see that on dividing by , we get a point where the remainder is zero and no more division is possible, Thus we get a finite number in the quotient which is the decimal expansion of the given rational number. Non Terminating means that while using the long division method we will not get a fixed number of digits in the quotient. In other words, while dividing the remainder does not become zero.

Admission Experiences.

The terminating decimal expansion means that the decimal representation or expansion terminates after a certain number of digits. About Us. Already booked a tutor? Learn Ncert All Solutions with tutors mapped to your child's learning needs. Learn Practice Download. Write down the decimal expansions of those rational numbers in Question 1 above which have terminating decimal expansions. In each case, decide whether they are rational or not.

Before going into a representation of the decimal expansion of rational numbers, let us understand what rational numbers are. So when these numbers have been simplified further, they result in decimals. Let us learn how to expand such decimals here. Examples: 6 , The real numbers which are recurring or terminating in nature are generally rational numbers. For example, consider the number It can be seen that the decimal part. Also the terminating decimals such as 0.

Decimal expansion of a rational number is terminating

Our terminating decimal calculator will teach you how to find the decimal representation of a number, detect the possible presence of repeating decimals , and much more. Keep reading to find out:. Additionally, we have prepared several examples of all the math explained in the text.

Tattoo wings on chest

Here, the process of long division goes on. Which one of the pie charts represents a terminating decimal number? If this condition is satisfied it means that the decimal expansion of the given rational number would be terminating. Maths Program. Commercial Maths. It is derived from the terminating decimal expansions. Here we get the remainder as zero and thus we terminate the division process at that time. The examples include rational numbers: whole numbers , integers, fractions of integers, and terminating decimals. Maths Games. Brain Teasers. Order decimals.

Rational numbers can be represented as decimals. The different types of rational numbers are Integers like -1, 0, 5, etc. The decimal representation of a rational number is converting a rational number into a decimal number that has the same mathematical value as the rational number.

Here as we are not getting the remainder 0 so we terminate the division and consider the quotient got so far. About Us. Please Login to comment You can suggest the changes for now and it will be under the article's discussion tab. Any number that can be stated as a fraction is rational. A terminating decimal expansion of a rational number means that while using the long division method we will get a fixed number of digits in the quotient. Year 11 Maths Worksheets. Create Improvement. How to find the Area of a Regular Polygon? Maximize your earnings for your published articles in Dev Scripter ! Correct answer is: it has an infinite number of digits after the decimal point A number is a non-terminating decimal number if it has an infinite number of digits after the decimal point. Enhance the article with your expertise. What kind of Experience do you want to share? Add Decimals. The rest are non-terminating decimals.