Angle of a sector

The area of sector of a circle is the space enclosed within the boundary of the sector. A sector always originates from the center of the circle.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. About About this video Transcript. A worked example of finding the area of a circle's sector using the area of the circle and the central angle of the sector.

Angle of a sector

In the circle above, the length of arc BC is degrees, and the segment AC is a diameter. What is the measure of angle ADB in degrees? Since we know that segment AC is a diameter, this means that the length of the arc ABC must be degrees. This means that the length of the arc AB must be 80 degrees. Since angle ADB is an inscribed angle, its measure is equal to half of the measure of the angle of the arc that it intercepts. This means that the measure of the angle is half of 80 degrees, or 40 degrees. What is the angle of a sector of area on a circle having a radius of? Now, to find the angle measure of a sector, you find what portion of the circle the sector is. Here, it is:. Now, multiply this by the total degrees in a circle:. Rounded, this is.

Example Question 31 : Circles. Evaluate the measure of.

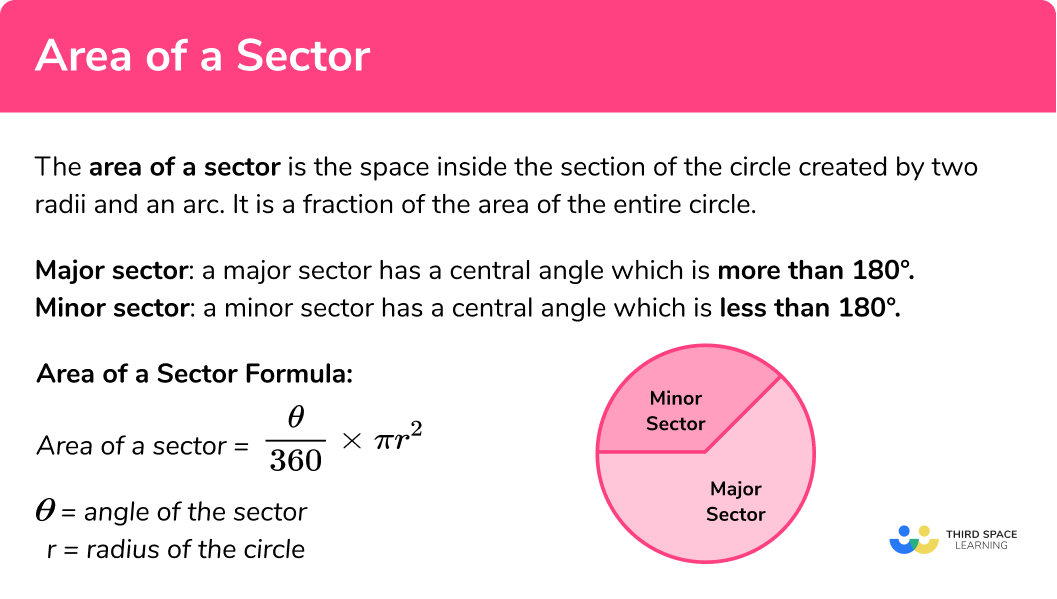
A sector of a circle is a portion or part of a circle that is composed of an arc and its two radii. You can compare the sector of a circle to the shape of a pizza slice. A sector is formed when two radii of the circle meet at both ends of the arc. An arc is simply a portion of the circumference of the circle. The definition of the sector of a circle in geometry can be given as the part of the circle enclosed by two radii and an arc of the circle. A sector of a circle is called the minor sector if the minor arc of the circle is a part of its boundary. It is the sector with a smaller area.

A circle has always been an important shape among all geometrical figures. There are various concepts and formulas related to a circle. The sectors and segments are perhaps the most useful of them. In this article, we shall focus on the concept of a sector of a circle along with area and perimeter of a sector. A sector is said to be a part of a circle made of the arc of the circle along with its two radii. It is a portion of the circle formed by a portion of the circumference arc and radii of the circle at both endpoints of the arc. The shape of a sector of a circle can be compared with a slice of pizza or a pie.

Angle of a sector

A pie has a diameter of 12". A piece is cut out, having a surface area of 4. What is the angle of the cut? This is simply a matter of percentages. We first have to figure out what percentage of the surface area is represented by 4.

The big greek

Privacy Policy. So, let us understand where the formula comes from. Sneha Kumar. If the sector angle is not given, but we know the arc length and the radius, the area of a sector can be calculated. Other lessons in this series include: Circles, sectors and arcs Area of a sector Perimeter of a sector Arc of a circle Parts of a circle. The formulas for both the measures of the circle are given by;. This topic is relevant for:. You can use the formulas of perimeter and area of a circle to make an equation. The part of a circle covered by 2 radii of a circle and their intercepted arc the arc coming in that portion is a sector of a circle. A minor sector is a sector that is less than a semi-circle, whereas, a major sector is a sector greater than a semi-circle. It is a portion of the circle formed by a portion of the circumference arc and radii of the circle at both endpoints of the arc. A sector always originates from the center of the circle. Practice Questions on Area of a Sector.

A sector of a circle is a portion or part of a circle that is composed of an arc and its two radii.

In this article, we will learn about what is a sector of a circle, formulas related to the sector of a circle along with solving a few examples on the sector of a circle. Identify Circles and Rectangles Game. Example 1: finding the area of a sector given the radius and angle Calculate the area of the sector shown below. But opting out of some of these cookies may affect your browsing experience. And then we just can solve for area of a sector by multiplying both sides by 81 pi. Since the arc S was one-fourth the circumference of the circle, the central angle formed by arc S should be one-fourth the total degrees of a circle. How to find the arc length of a sector of a circle? If compartment 1 is at 0 degrees and Eric enters compartment 13, what angle is he at? Formulas of Sector of a Circle 3. Updated November 03, How can we find the area of a sector of a circle when the central angle is not given?