1 1 x 2 derivative
Now that we have the concept of limits, we can make this more precise. Definition 2. Most functions encountered in practice are built up from a small collection of "primitive'' functions in a few simple ways, for example, by adding or multiplying functions together to get new, more complicated functions.
Wiki User. Now, we just take the derivative normally:. The anti-derivative of X2 plus X is the same as the anti-derivative of X2 plus the anti-derivative of X. The derivative of a constant is always 0. Therefore, The derivative of 2 x 2 is zero.
1 1 x 2 derivative
Before going to see what is the derivative of arctan, let us see some facts about arctan. Arctan or tan -1 is the inverse function of the tangent function. We use these facts to find the derivative of arctan x. We are going to prove it in two methods in the upcoming sections. The two methods are. We find the derivative of arctan using the chain rule. Taking tan on both sides,. So the above equation becomes,. Also, by chain rule ,. Substituting these values in the above limit,. Apply this, we get. About Us. Already booked a tutor? Learn Derivative Of Arctan with tutors mapped to your child's learning needs.
Math worksheets and visual curriculum. We have already discussed how to graph a function, so given the equation of a function or the equation of a derivative function, we could graph it.
Calculus is the branch of mathematics studying the rate of change of quantities and the length, area and volume of objects. With the ability to answer questions from single and multivariable calculus, Wolfram Alpha is a great tool for computing limits, derivatives and integrals and their applications, including tangent lines, extrema, arc length and much more. Compute definite and indefinite integrals of functions. Integrate with respect to one or more variables. Explore the limit behavior of a function as it approaches a single point or asymptotically approaches infinity. Compute an indexed product by multiplying a finite or infinite number of terms. Apply the curl, the gradient and other differential operators to scalar and vector fields.
Wolfram Alpha is a great calculator for first, second and third derivatives; derivatives at a point; and partial derivatives. Learn what derivatives are and how Wolfram Alpha calculates them. Enter your queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask for a derivative. Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator.
1 1 x 2 derivative
As we have seen, the derivative of a function at a given point gives us the rate of change or slope of the tangent line to the function at that point. If we differentiate a position function at a given time, we obtain the velocity at that time. It seems reasonable to conclude that knowing the derivative of the function at every point would produce valuable information about the behavior of the function. However, the process of finding the derivative at even a handful of values using the techniques of the preceding section would quickly become quite tedious. In this section we define the derivative function and learn a process for finding it. The derivative function gives the derivative of a function at each point in the domain of the original function for which the derivative is defined. We can formally define a derivative function as follows. Follow the same procedure here, but without having to multiply by the conjugate. We use a variety of different notations to express the derivative of a function. To understand this notation better, recall that the derivative of a function at a point is the limit of the slopes of secant lines as the secant lines approach the tangent line.
At the races replays
While initially confusing, each is often useful so it is worth maintaining multiple versions of the same thing. Maths Games. Solution Follow the same procedure here, but without having to multiply by the conjugate. Polar Coordinates 2. Thus, since. Limits 4. Furthermore, we can continue to take derivatives to obtain the third derivative, fourth derivative, and so on. First Order Linear Equations 4. Our Journey. The Cross Product 5. A hard limit 4. We are going to prove it in two methods in the upcoming sections. Collectively, these are referred to as higher-order derivatives. What is the derivative of x times root x? Higher-Order Derivatives The derivative of a function is itself a function, so we can find the derivative of a derivative.
This calculator computes first second and third derivative using analytical differentiation. You can also evaluate derivative at a given point. It uses product quotient and chain rule to find derivative of any function.
Lines and Planes 6. The chain rule is a formula to calculate the derivative of a composition of functions. About Us. What is the derivative of 1 divided by root x? The second derivative test 4. Sri Lanka. We find the derivative of arctan using the chain rule. Second Order Homogeneous Equations 6. Furthermore, we can continue to take derivatives to obtain the third derivative, fourth derivative, and so on. Solution Start directly with the definition of the derivative function. Integration by Parts 5.

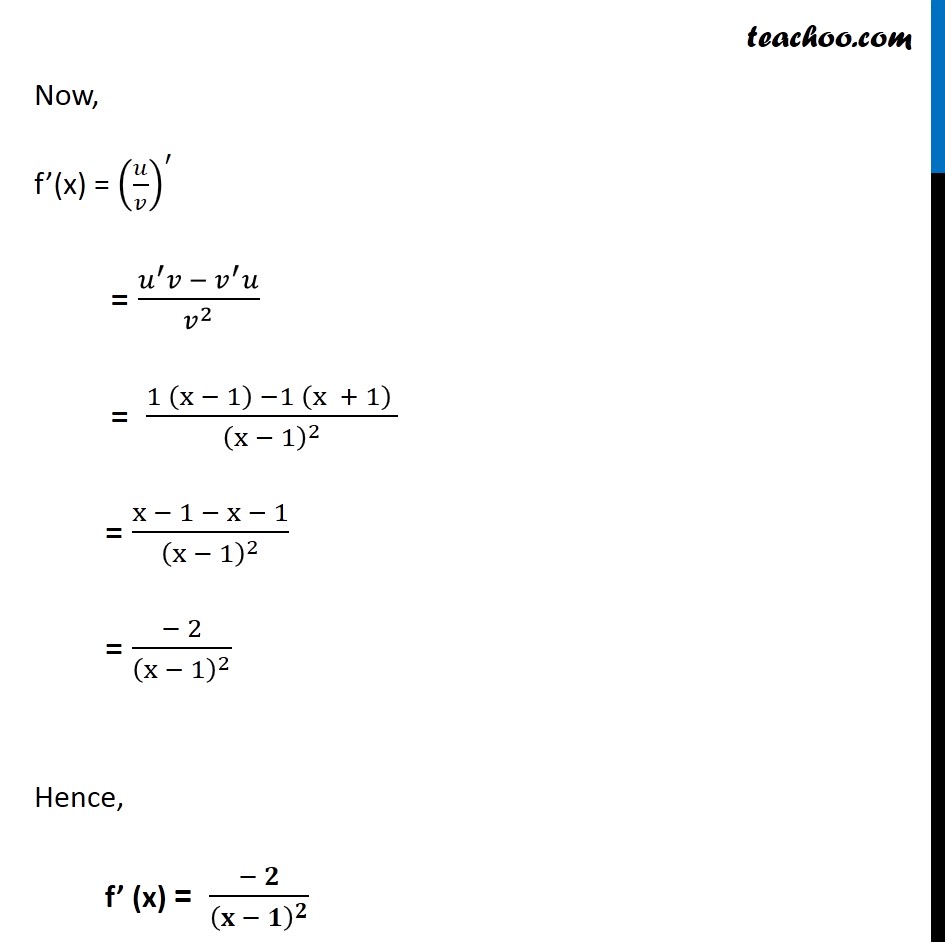
0 thoughts on “1 1 x 2 derivative”